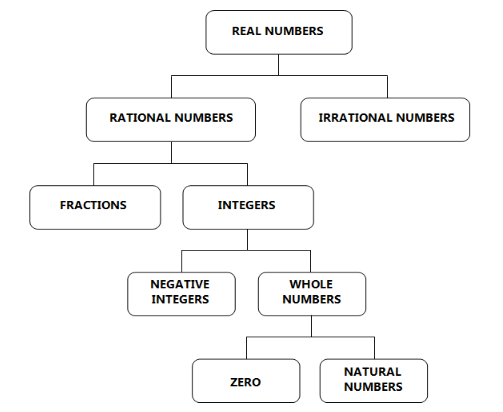
R = Real Numbers:
All rational and irrational numbers are called real numbers.
I = Integers:
All numbers from (…-3, -2, -1, 0, 1, 2, 3…) are called integers.
Q = Rational Numbers:
Real numbers of the form p/q, q ≠ 0, p, q ∈ I are rational numbers.
All integers can be expressed as rational, for example, 5 = 5/1
Decimal expansion of rational numbers terminating or non-terminating recurring.
Q’ = Irrational Numbers:
Real numbers which cannot be expressed in the form p/q and whose decimal expansions are non-terminating and non-recurring.
Roots of primes like √2, √3, √5 etc. are irrational
N = Natural Numbers:
Counting numbers are called natural numbers. N = {1, 2, 3, …}
W = Whole Numbers:
Zero along with all natural numbers are together called whole numbers. {0, 1, 2, 3,…}
Even Numbers:
Natural numbers of the form 2n are called even numbers. (2, 4, 6, …}
Odd Numbers:
Natural numbers of the form 2n -1 are called odd numbers. {1, 3, 5, …}
Why can’t we write the form as 2n+1?
Remember this!
All Natural Numbers are whole numbers.
All Whole Numbers are Integers.
All Integers are Rational Numbers.
All Rational Numbers are Real Numbers.
Prime Numbers:
The natural numbers greater than 1 which are divisible by 1 and the number itself are called prime numbers, Prime numbers have two factors i.e., 1 and the number itself For example, 2, 3, 5, 7 & 11 etc.
1 is not a prime number as it has only one factor.
Composite Numbers:
The natural numbers which are divisible by 1, itself and any other number or numbers are called composite numbers. For example, 4, 6, 8, 9, 10 etc.
Note: 1 is neither prime nor a composite number.
Euclid’s Division lemma:
Euclid’s Division lemma:
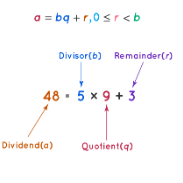
Euclid’s division algorithm, as the name suggests, has to do with divisibility of integers. Stated simply, it says any positive integer a can be divided by another positive integer b in such a way that it leaves a remainder r that is smaller than b.
In other words,Given two positive integers a and b, there exist unique integers q and r satisfying a = b q + r, 0 ≤ r ≤ b
Notice this. Each time ‘r’ is less than b. Each ‘q’ and ‘r’ is unique.
Many of you probably recognise this as the usual long division process. Although this result is quite easy to state and understand, it has many applications related to the divisibility properties of integers. We touch upon a few of them, and use it mainly to compute the HCF of two positive integers.
Application of lemma:
Euclid’s Division lemma is used to find HCF of two positive integers. Example: Find HCF of 24 and 56
Apply lemma to 24 and 56.
Take bigger number and locate ‘b’ and ‘r’. 56 = 24 × 2 + 8
Since 8 ≠ 0, consider 24 as the new dividend and 8 as the new divisor. 24 = 8 × 3 + 0
Since the remainder is zero, divisor (8) is HCF.
Although Euclid’s Division lemma is stated for only positive integers, it can be extended for all integers except zero, i.e., b ≠ 0.
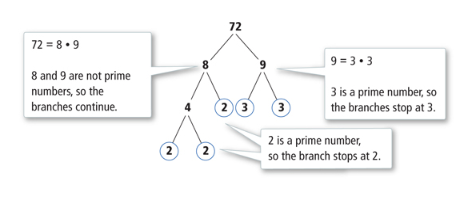
II. Constructing a factor tree
Write the number as a product of prime number and a composite number
Example:
Factorize 72
Repeat the process till all the primes are obtained
∴ Prime factorization of 72 = 23 x 32
Fundamental Theorem of Arithmetic:
Fundamental Theorem of Arithmetic:
The Fundamental Theorem of Arithmetic, on the other hand, has to do something with multiplication of positive integers. You already know that every composite number can be expressed as a product of primes in a unique way—this important fact is the Fundamental Theorem of Arithmetic. In other words, Every composite number can be expressed as a product of primes, and this expression is unique, apart from the order in which they appear.
Again, while it is a result that is easy to state and understand, it has some very deep and significant applications in the field of mathematics. We use the Fundamental Theorem of Arithmetic for two main applications.
Applications:
I. To prove the irrationality of numbers.
The sum or difference of a rational and an irrational number is irrational.
The product or quotient of a non-zero rational number and an irrational number is irrational.
II. To determine the nature of the decimal expansion of rational numbers
Let x = pq, p and q are co-primes, be a rational number whose decimal expansion terminates. Then the prime factorization of q’ is of the form 2m5n, m and n are non-negative integers.
Let x = pq be a rational number such that the prime factorization of ‘q’ is not of the form 2m5n, ‘m’ and ‘n’ being non-negative integers, then x has a non-terminating repeating decimal expansion.
 Discuss
Discuss